Moncef Hidane completed a post-doctoral 2013-2014 under the Labex CPU. He previously completed a doctoral thesis at the laboratory GREYC in Caen, the decomposition problem of graphs of signals. He is currently research professor at INSA Loire Valley.

The topic of the post-doc concerns the 3D image reconstruction from a low-resolution full acquisition and partial acquisition in very high resolution. So this is a super-resolution inverse problem.

The model adopted for the low resolution acquisition is that of a convolution operator followed by a spatial or spectral sub-sampling. Initially, the impulse response of the sensor is assumed to be known. An additive white noise is integrated into the model.

The model adopted for the high resolution acquisition is one of an occlusion in which the support is known. An additive white noise is also integrated into the model.

The first track is followed by that of a regularization using an a priori isotropically total variation. Two versions are contemplated: one with inequality constraints, on the other hand with equality constraints. In this context, an important part of the work concerns minimization criteria in each case. Due to the non-differentiability of the measure total variance algorithms from the non-smooth convex optimization are considered. The goal is to have fast and robust algorithms for computing solutions.

The second track is followed by an adjustment using a non-local operator. The idea is to take advantage of both the volume low resolution and high resolution images to construct a priori regularization type nonlocal total variation.

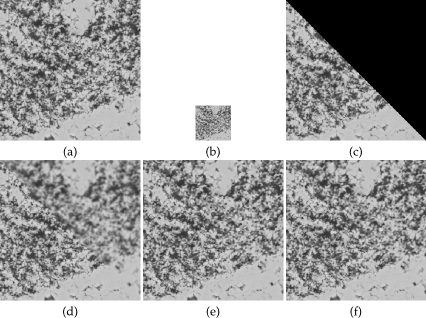
The following figure illustrates the problem and the results obtained with the two previous approaches.

(a) image originale 256 x 256; (b) image BR (facteur 4); (c) image HR partielle; (d) interpolation bicubique de (b) (psnr=24.43); (e) reconstruction TV (psnr=29.52); (f) recontruction par régularisation non locale (psnr=30.16).